 As discussed previously, there are lessons aplenty to learn from the ongoing Ebola outbreak in West Africa. One simple lesson is this: Even with a low basic reproduction ratio (symbolized mathematically as R0), a pathogen can still spread widely under the right conditions.
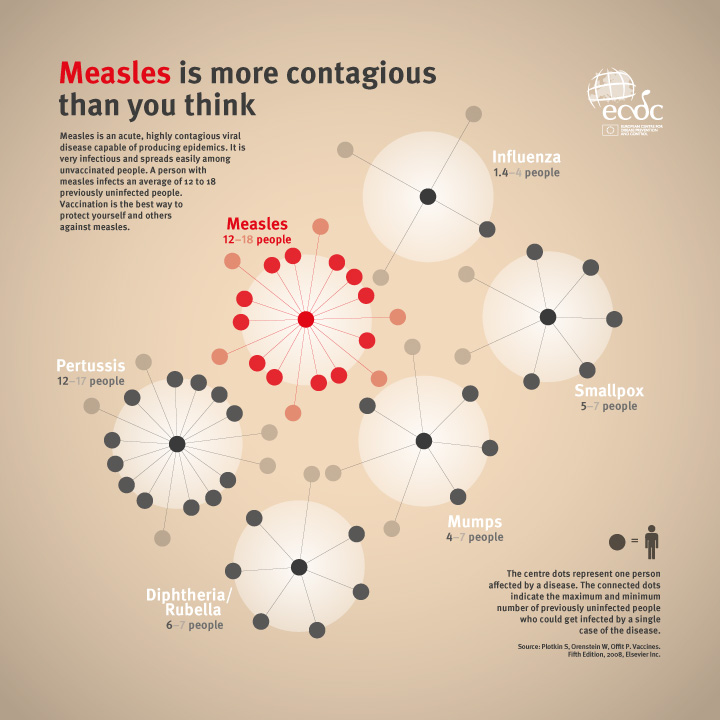
As discussed previously, there are lessons aplenty to learn from the ongoing Ebola outbreak in West Africa. One simple lesson is this: Even with a low basic reproduction ratio (symbolized mathematically as R0), a pathogen can still spread widely under the right conditions. Current estimates of the basic reproduction number for this Ebola outbreak are roughly in the range of 1.3-2.5. That's pretty modest when compared with other notorious agents. Estimates of R0 for smallpox outbreaks, for example, were typically 4-10 and those for cholera epidemics can be in the range of 3-12. Measles outbreaks can have R0 values of up to 18.
R0 itself is sometimes thought of as a surrogate for "epidemic potential". Certainly, and by definition, pathogens with high R0 spread quickly, whereas pathogens with lower R0 don't. Does this mean that pathogens possessing relatively low values of R0 have lower potential to harm public health?
Certainly not. In fact, pandemic influenza viruses, for example, often fall in the range 1.5-2.0. Pathogens associated with lower values of R0 can spread widely if control efforts are not effective. In the case of pandemic influenza, control measures include vaccination and handwashing. In the current Ebola situation, due to a range of social, economic, and political factors, it has been difficult to implement widespread, effective control measures. The infection has thus spread and will likely continue do so in the region.
Does this have implications for other infections? It absolutely does. One could imagine some theoretical pathogen, for example, that is spread predominantly by hands (call it "pathogen X") and that is not killed by alcohol based hand rub (ABHR). Then, in circumstances where ABHRs are used in place of handwashing, one might imagine that pathogen X could, over time, become widely prevalent, even if it does not possess a high R0. Pathogen X might be similar to Clostridium difficile; one estimate of R0 for C. difficile is in the range 0.5-1.5.
For this reason, we should not think only in terms of R0 for classifying pathogens as dangerous or not. While high R0 pathogens spread quickly, leaving little time to react and take action, Ebola in West Africa this year demonstrates that a pathogen possessing a more modest R0 can result in a dangerous public health situation.
There's a story, which is possibly apocryphal, that Enrico Fermi once remarked that nowhere is it written that the laws of physics must be linear. I think there's an analogue that should be kept in mind in infectious disease epidemiology: Nowhere is it written that dangerous pathogens must have high R0.
(image source: ECDC)
Do you have any feel for where and when the term "basic reproduction number" originated in epidemiology? Seems a very poor choice given that it refers simply to the rate of spread of cases in a given population, which is very context dependent.
ReplyDeleteIt is contextual, and so it's not strictly speaking correct to attribute one R0 to any given pathogen. I believe the historical development of the concept in infectious disease epidemiology grew out of malaria modeling (where, as I recall, the original symbolism was Z, not R) in the first half of the 20th Century. Authoritative sources on the history and subtleties of the concept can be found in https://people.ifm.liu.se/unwen/usaresa%20feb%202011/artiklar/referenser/R0article2002.pdf and http://rsif.royalsocietypublishing.org/content/2/4/281.full. I myself have a love-hate relationship with R0 -- we often ask an awful lot from a single metric, which is itself often difficult to compute and interpret (http://www.hindawi.com/journals/cmmm/2011/527610/). That all said, it is important and hugely useful.
DeleteGreat, thanks very much David, I will try to read those, especially the first one, where it appears Heesterbeek discusses the concept in relation to the "intrinsic rate of increase" (r) in popln. biology, a topic which interests me. But it all looks helpful.
Delete